Copyright, Burger & Brown Engineering, Inc., August 14, 2018
Written by Philip M. Burger, P.E. Emeritus
Phil Burger is the founder of Burger & Brown Engineering, Inc. in Grandview, MO and currently serves as Scientific Cooling Instructor.
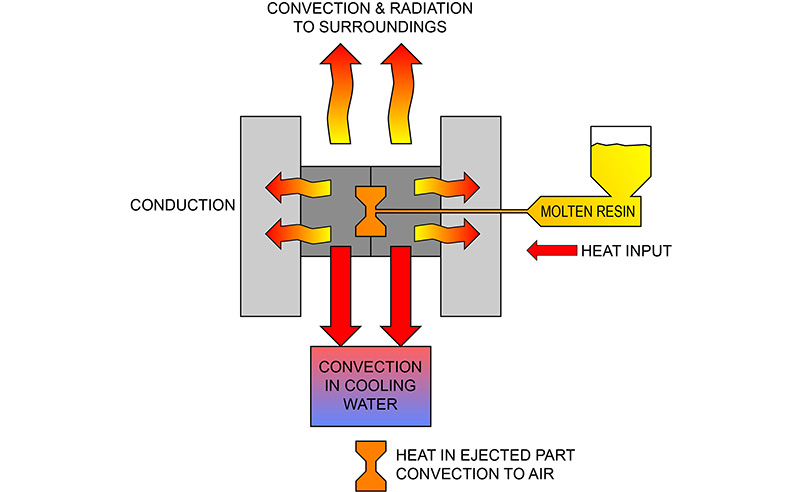
This page is designed to show the calculation steps to figure heat transfer from an injection mold through the clamp plate and into the platen.
Variables
ΔT = Temperature difference between hot and cold side of a plate
R = Resistance = L/(K x A)
A = surface area of plate
K = heat transfer coefficient (BTU/hr/Ft2/in./ ΔT)
L = plate thickness, ft.
Assume a 12” square P-20 steel clamp plate with K = 16.8, Area = 1 sq.ft., thickness = 1.875”, Call this value R1 as follows:
L = 1.875/12 = .149 ft.
A = 1 sq.ft.
Resistance R1 = .149 ft./(16.8 x 1 sq.ft.) = .0089
If the A-plate of the mold is kept at 175°F by a mold temperature control and the clamp plate is clamped to the machine platen which might be around 90°F we can estimate that ΔT (between the hot and cool sides of the clamp plate) = 85°F
Heat Transfer Rate, Q = ΔT/R1 = 85/.0089 = 9551 BTU/hr.
Next, for an insulator board 1/2” thick we can calculate an R2 value as follows: L = .50”/12/in/ft = .042 ft.. The heat transfer coefficient of the insulator board is 1.9, so the math goes like this:
Resistance R2 = .042/(1.9 x 1) = .022
With the ½” insulator board added the calculation is as follows:
Heat Transfer Rate, Q = ΔT/(R1 + R2) = 85/(.0089 + .022) = 85/.031 = 2742 BTU/hr.
SUMMARY
Without the insulator board, mold at 175°F, heat transfer through the clamp plate and into the platen is approximately:
Q = ΔT/R1 = 85/.0089 = 9551 BTU/hr
With a ½” insulator board with a heat transfer coefficient of 1.9 the heat transfer rate to the platen is approximately:
Q = ΔT/(R1 + R2) = 85/(.0089+.022) = 85/.031 = 2742 BTU/hr.
Savings is 6809 BTU/hr.
This change results in lower energy cost and greater process stability.
